brandsvast.blogg.se

1. #BEST EXPLANATION OF GEOMETRIC AND ARITHMETIC SEQUENCES HOW TO#
2. #BEST EXPLANATION OF GEOMETRIC AND ARITHMETIC SEQUENCES PLUS#
3. #BEST EXPLANATION OF GEOMETRIC AND ARITHMETIC SEQUENCES SERIES#

Well for an arithmeticĪmount regardless of what our index is. That we're adding based on what our index is. So this looks close,īut notice here we're changing the amount

#BEST EXPLANATION OF GEOMETRIC AND ARITHMETIC SEQUENCES PLUS#

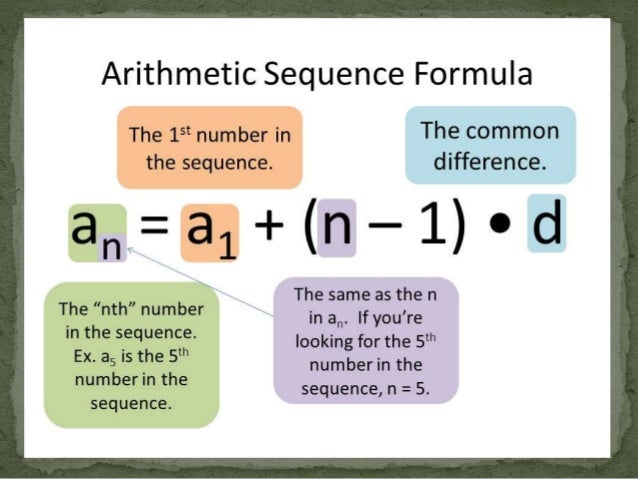
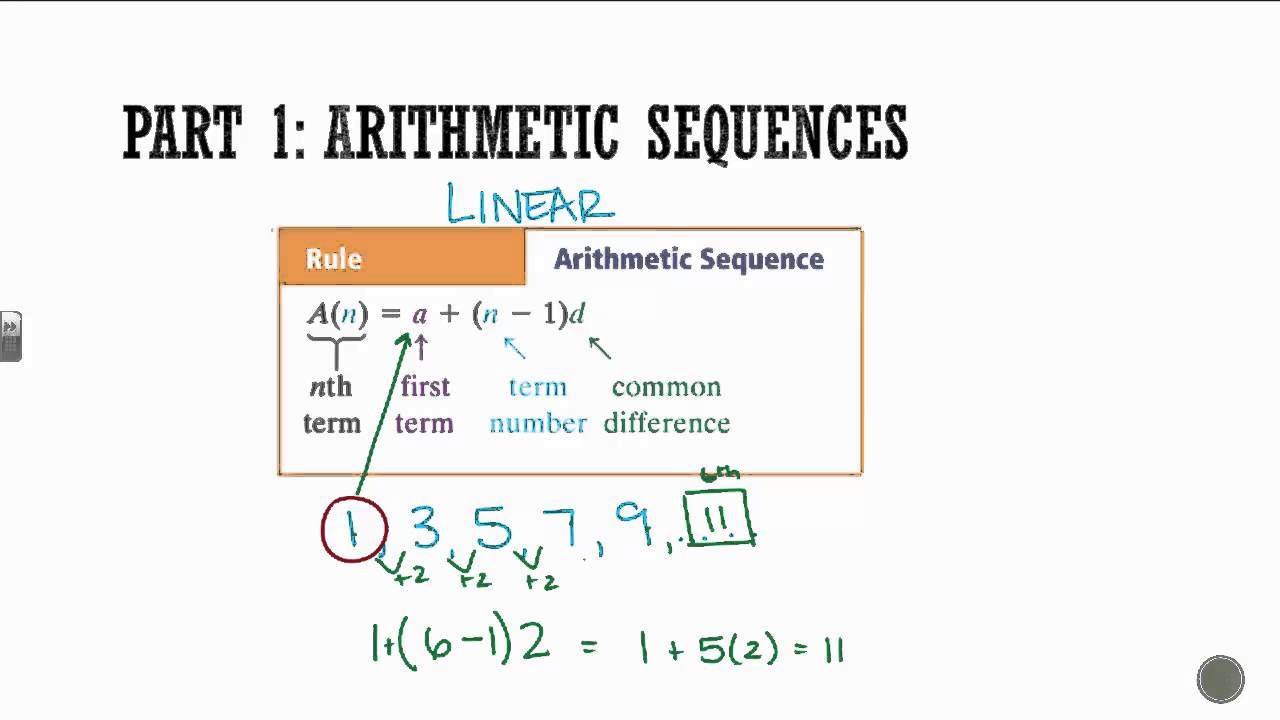
Previous term plus whatever your index is. Or greater, a sub n is going to be equal to what? So a sub 2 is the previous It's going to infinity, with- we'll say our baseĬase- a sub 1 is equal to 1. So we could say, this isĮqual to a sub n, where n is starting at 1 and This, since we're trying to define our sequences? Let's say we wanted toĭefine it recursively. So this, first of all,Īrithmetic sequence. We're adding a differentĪmount every time. Giveaway that this is not an arithmetic sequence. Is is this one right over here an arithmetic sequence? Well, let's check it out. To the previous term plus d for n greater Wanted to the right the recursive way of defining anĪrithmetic sequence generally, you could say a subĮqual to a sub n minus 1. And in this case, k is negativeĥ, and in this case, k is 100. That's how much you'reĪdding by each time. So this is one way to defineĪn arithmetic sequence. Number, or decrementing by- times n minus 1. Between successive words, there is a common difference. A geometric sequence is a collection of integers in which each subsequent element is created by multiplying the previous number by a constant factor. If you want toĭefine it explicitly, you could say a sub n isĮqual to some constant, which would essentiallyĬonstant plus some number that your incrementing- Arithmetic Sequence is a set of numbers in which each new phrase differs from the previous term by a fixed amount. Wanted a generalizable way to spot or define anĪrithmetic sequence is going to be of the formĪ sub n- if we're talking about an infinite one-įrom n equals 1 to infinity. Than 1, for any index above 1, a sub n is equal to the In geometric sequences, all numbers have a common ratio, meaning that the quotient of each member and the one before it is some constant value.

#BEST EXPLANATION OF GEOMETRIC AND ARITHMETIC SEQUENCES SERIES#

One definition where we write it like this, or weĬould write a sub n, from n equals 1 to infinity. The geometric mean is best used to calculate the average of a series of data where each item has some relationship to the others. To define it explicitly, is equal to 100 plus Of- and we could just say a sub n, if we want

Is the sequence a sub n, n going from 1 to infinity So this is indeed anĬlear, this is one, and this is one right over here. Is this one arithmetic? Well, we're going from 100. The arithmetic sequence that we have here.

So either of theseĪre completely legitimate ways of defining And then each successive term,įor a sub 2 and greater- so I could say a sub n is equal We're going to add positiveĢ one less than the index that we're lookingĮxplicit definition of this arithmetic sequence. So for the secondįrom our base term, we added 2 three times. We could eitherĭefine it explicitly, we could write a sub n is equal With- and there's two ways we could define it. So this is clearly anĪrithmetic sequence. Then to go from negativeġ to 1, you had to add 2. These are arithmetic sequences? Well let's look at thisįirst one right over here. In this unit, we'll see how sequences let us jump forwards or backwards in patterns to solve problems. Term is a fixed amount larger than the previous one, which of Sequences are a special type of function that are useful for describing patterns.

So first, given thatĪn arithmetic sequence is one where each successive The index you're looking at, or as recursive definitions. And then just so thatĮither as explicit functions of the term you're looking for, Out which of these sequences are arithmetic sequences.

Term is a fixed number larger than the term before it.

#BEST EXPLANATION OF GEOMETRIC AND ARITHMETIC SEQUENCES HOW TO#

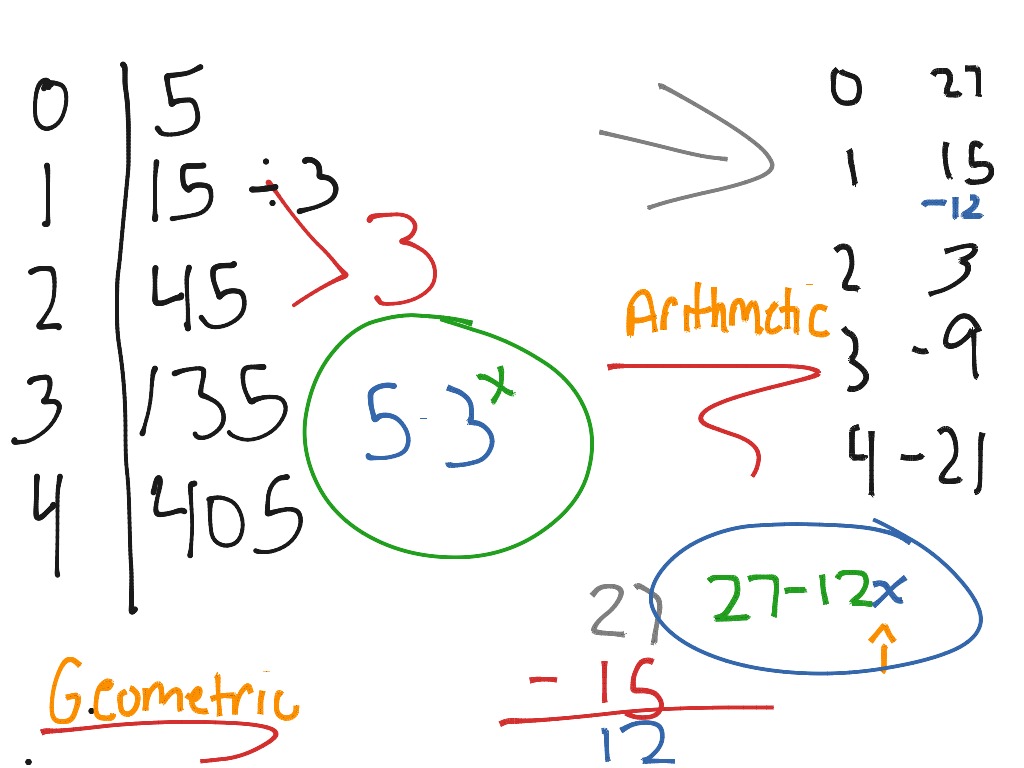
Then we will investigate different sequences and figure out if they are Arithmetic or Geometric, by either subtracting or dividing adjacent terms, and also learn how to write each of these sequences as a Recursive Formula.Īnd lastly, we will look at the famous Fibonacci Sequence, as it is one of the most classic examples of a Recursive Formula.Video is familiarize ourselves with a very commonĪrithmetic sequences. I like how Purple Math so eloquently puts it: if you subtract (i.e., find the difference) of two successive terms, you’ll always get a common value, and if you divide (i.e., take the ratio) of two successive terms, you’ll always get a common value. Then, we either subtract or divide these two adjacent terms and viola we have our common difference or common ratio.Īnd it’s this very process that gives us the names “difference” and “ratio”. And adjacent terms, or successive terms, are just two terms in the sequence that come one right after the other. Well, all we have to do is look at two adjacent terms. It’s going to be very important for us to be able to find the Common Difference and/or the Common Ratio. Comparing Arithmetic and Geometric Sequences